北京师范大学系统科学学院-yb亚博全站首页

keep-left behavior induced by asymmetrically profiled walls

c. l. n. oliveira,1, a. p. vieira ,d. helbing, j. s. andrade,

jr .h. j. herrmann

(physical review x 6, 011003 (2016))

这篇文章在计算和理论上分析并证明了：不对称的墙可以组织人群在一个走廊上朝着相反的方向运动。并且通过设置参数，可以精确地使得人群总是走左边或者右边。这种现象受到几个因素的影响：走廊轮廓的不对称性，走道宽度，人群的流速和密度，噪音干扰的强度。文章深度研究了这些参数的对人流的影响，并且找出了从无序到有序的一个相变点。通过研究发现，只有人流速度在很小的范围内变化时才会出现有序的状态；尽管噪音会对抑制有序人流的形成，但是人流还是能持续保持在有序状态，这与“通过加热而冻结”现象相反，那么说明锯齿状的走廊不仅可以使得人群“靠右走”，还可以在防止人群因为噪音拥堵。该发现可以用于微流体的控制以及人群状态的转换。

in the present work, we show that corridors with asymmetrically profiled walls can induce organization in the flow of pedestrians. precisely, the key element of our approach is to use walls having the geometry of a ratchet, in the present work, we show that corridors with asymmetrically profiled walls can induce organization in the flow of pedestrians. precisely, the key element of our approach is to use walls having the geometry of a ratchet, i.e., a zigzag structure such as the one shown in fig. 1(a).we consider that all pedestrians (modeled here as self-driven particles) in the channel are identical except that half of them are downward movers, i.e., driven by a force downward, while the other half are upward movers, i.e., driven by a force upward. these forces try to impose a constant drift velocity. a repulsive potential acts between particles and with the walls, while a stochastic force introduces disorder. the flow orientation along the walls strongly depends on the system’s parameters. moreover, as we will see later, increasing the strength of the disorder may suppress organization.

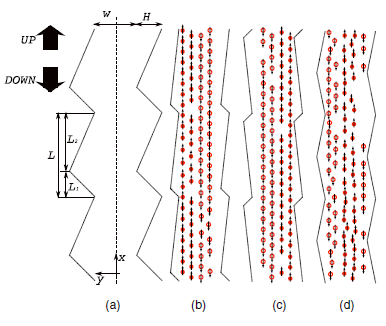
fig. 1. channel geometry and final configurations of pedestrians for different asymmetry parameters. (a) channel, of depth h and width w, through which a preferred flow orientation of particles is induced. the meaning of geometric parameters is indicated. (b–d) typical configurations in the steady state, where the particles are keeping left in asymmetric wall channels but are mixed in symmetric ones. upward movers (downward movers) are represented by full (hollow) particles. the velocity of each particle is indicated by arrows.

in fig. 2, we show, for different drift velocities in systems without noise, how the degree of asymmetry b changes the lanes and induces preferential sides of the particle flows in the channel. interestingly, the larger , the more abrupt the change between the ordered states, , as b changes. for large positive values of b, we find; i.e., everybody is keeping left perfectly. for large negative values of b, we find , corresponding to everybody keeping right. finally, there is a mixed state for b close to zero, where no preferred side is observed.

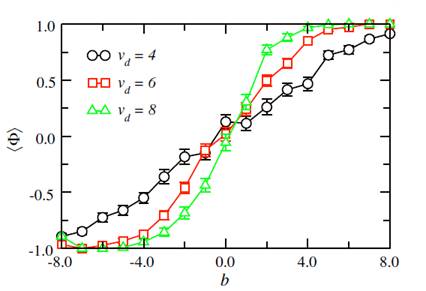
fig. 2. average order parameter  as a function of the asymmetry parameter b, for three different values of the drift velocity. we see that order is only induced for > 0. the slower, the highershould be in order to achieve a two-lane pattern. the remaining parameters, including the lack of noise, are the same as in fig. 1.

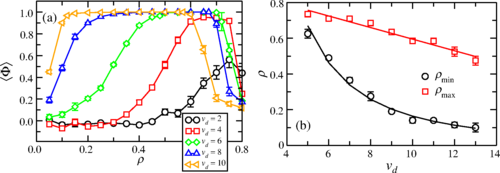
fig.3 dependence of density on drift velocity for ordered states. in panel (a), the average order parameter ⟨φ⟩ is shown as a function of the density for θ=0, b=8, h=2, and several values of the drift velocity vd. in panel (b), we show the maximum (red squares) and minimum (black circles) densities for which ⟨φ⟩=1, as functions of the drift velocity. a power-law fit for the minimum density yields ρmin=17.6v−2.03d, whereas a linear fit for the maximum density gives ρmax=0.919−0.032vd. the equations extracted from the fits meet at two points, namely, vd≈4.67 and vd≈28.09.

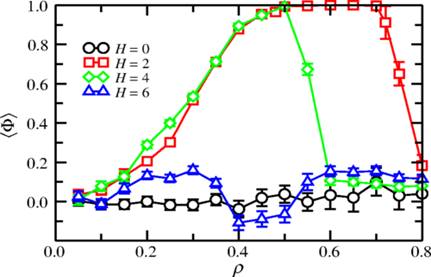
fig. 4. average order parameter as a function of the particle density, for θ=0, b=8, vd=6, and various values of the depth hof the serrated region.

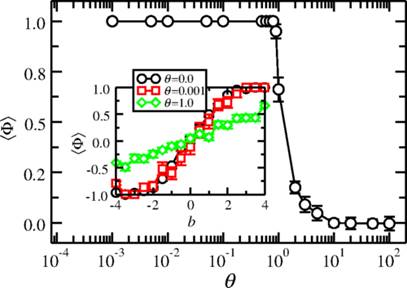
fig. 5.average order parameter as a function of θ, for ρ=0.6, b=8, vd=6, and h=2. the inset shows the crossover from ⟨φ⟩=−1 to ⟨φ⟩=1 as b changes from −8 to 8, for different values of θ.

原文链接：

推荐者：郑雅婷